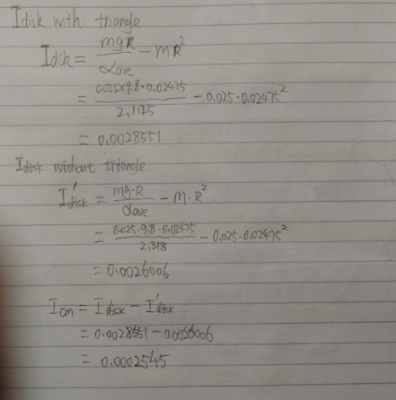May - 13 - 2015 Lab18 : Finding the Moment of Inertia of a Uniform Triangle Rotating about it's Center of Mass

Lab 18

Purpose: To determine the moment of inertia of a right triangular thin plate around it's center of mass, for two perpendicular orientations of the triangle.

How to find out moment of inertia of right triangular is what about of this lab. I am going to use one specify equipment to do it today.
Why we chose this equipment because it can give the angular acceleration of the disk. By knowing the angular acceleration , we can use the formula I = a * r calculate the moment of inertia. Thus, The moment of inertia of triangular is the moment of inertia of disk with triangular minus the moment of inertia of disk without the triangular.

Apparatus: 

Connecting the top disk to computer only and set the disk will spin independently and bottom one stops. 

Change the disk to 200 counts per rotating.  

weight of disk is 1.36 kg

radius of disk is 0.0625 m

hanging mass is 0.025 kg

Large pulley radius is 0.0495 m.

Triangular long side b = 0.1485 m
Triangular short side a = 0.098 m
weight of triangular 0.455 kg
Those data will be used on next steps.

turn on the air so the top disk can rotate, then Start collect on the computer and record the radius and distance on computer.

Those are two experiment should be down, one triangular is at right, one is at bottom. 

Part 1

Part 1 is to find out the moment of inertia with those two pose around it's center of mass.

To find out the moment of inertia.

do three experiment, one is without triangular, one is with triangular, and another one is with triangular with different face. 

Record the up and down angular acceleration. 

Because disk have friction, so up and down accelerations will be different.

By using this equipment, we can find the Angular acceleration up and down with three different condition. 

And then use them to find the moment of inertia of disk with those different conditions.

That's equation we use to find out the moment of inertia.
Why we need to find out the moment of inertia is that The moment of inertia of triangular is the moment of inertia of disk with triangular minus the moment of inertia of disk without the triangular.
so we can find the uniform triangular by using this method.

Then collect the data.

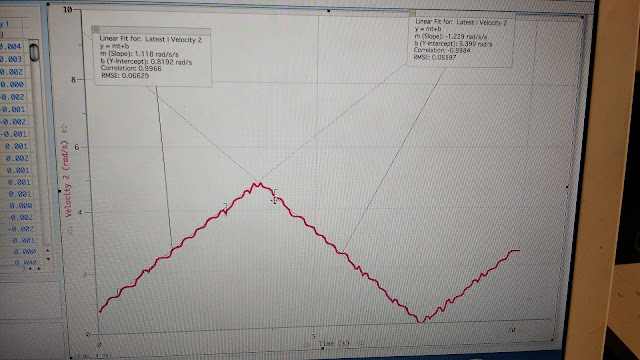
Those graphs are the one without triangular and one with triangle with long side pointing up.

They are angular velocity vs time graph. So the slope of the line are angular acceleration.

Plug the data into the function which can find the moment of inertia

After calculate, moment of inertia of disk with triangular is 0.0028551 kg*m^2

the moment of inertia of disk without the triangular is 0.0026006

Therefore, moment of inertia of triangular rotating aroung the center of mass is 0.0002545 kg * M ^2







Using the same method, find out the another condition's moment of inertia of triangular. 

So the moment of inertia of triangular in this pose is 0.0054888 kg* m^2.

In part 1, I obtain the two different moment of inertia around it's center of mass which are 0.0054888 kg* m^2 and 0.0002545 kg * M ^2.

Part 2

Part two is to test our results.

If without the experiment, it's very difficult to find out the moment of inertia around it's center of mass, so need to change the way to calculate them.

If not around the center of mass but around one side.

By around the one edge of the triangular.







it's easy to find I paralle axis and M(d).; thus, the moment of inertia around it's center of mass is just I paralle axis - M(d)






To find I paralle axis and M(d). 

After calculation
Moment of inertia around center of mass is just the （1/18) *M* a^2 for this

another will be （1/18) *M* b^2

So for the first one

Moment of inertia around center of mass is 0.000242768 kg* m^2 
Uncertainty compare to the value got on the experiment is 4.8 % off.


Another one:

Moment of inertia around center of mass is 0.000557432 kg* m^2 
Uncertainty compare to the value got on the experiment is 1.53 % off.

Conclusion:

This is the lab about how to find out the moment of inertia of a uniform triangular rotating about it's center of mass. By using formula and measurement, we find the experiment value and compare with the prediction value to see how well the results are. With friction, mistakes, and not advanced equipment our results are off by 1.53% and 4.8% are good results. 

May-13-2015 Lab17: Moment of Inertia and Frictional Torque

Lab 17

Purpose of this lab is to find the moment of inertia and frictional torque on a spinning metal disk.

To do this lab:

Appropriate:

Set up equipment like the picture shown.

First: 

Determine the rotating part of apparatus's moment of inertia.
Because the rotating part has three parts. Two same cylinders and one disk.

So to find the moment of inertia of total is measure the radius of them and weight of them and add each moment of inertia together.

it's easy to find the total mass of the rotating parts, so using this to find the weight of each part.

 

Those are the data after calculation.

Radius of the disk and cylinders, mass of disk and cylinders.

On the right is how to calculate the Moment of inertia total. (Knowing the moment of inertia of cylinder and disk are 1/2 * m * r^2 if they rotate like this lab.)

Second:

Determine it's angular deceleration as it slows down, and calculate the frictional torque acting on the apparatus. 

(Because it has friction when rotating, so the deceleration acceleration is caused by friction)

To find the angular deceleration of the disk is to rotate it and using the video capture to get the velocity of a specific point on the disk. then find the acceleration, then find the angular acceleration.

Using the video capture to video the whole process of the disk slow down.

Make sure mark an obvious sign so it's easy to track where the mark went.

Follow the mark and point the mark position.

Set the Center of mass of this disk is the origin.

The disk radius as  measurement  which is 0.10025 meter

This is how the graph looks like. 

Red is the position on X axis

and  Velocity on x axis can be found.

Blue is the position on Y axis.

and  Velocity on y axis can be found.

To find the angular deceleration, set up a new column called Velocity total (Vt) which is (Vx^2 + Vy^2)^0.5

Then show the graph Vt vs time graph and linear fit a line and get the angular deceleration.

the slope of the line is the deceleration which is - 0.05329 m/s^2.

Using the formula a = alph * r
get the angular deceleration is -0.533rad /s

the torque is the
T = I * alph
T= -0.01063 N *m

Third:

Checking our data in previous.

Connecting the apparatus to a 500 -gram's cart, let the cart roll down an inclined track with some angle for distance 1 meter. Calculate how long it take  to move 1 meter from the rest.

Doing this is to compare the time by clock with the time by calculating.

Which can be find from clock.

After three experiments which are let the cart go distance 1 meter and record the time and get the average time which is 7.2 second.

To check the data should to derive a equation compare the time on experiment value and the real time measure by clock.

Draw a force graph, and analyse the force.

Three equations, Know that angle which is 48 degree measured, m is 499 grams, Torque friction is 0.01063 N* m, I-total =0.02 kg*m^2, radius of the small disk is 0.01575 m. Three unknown are T, angular acceleration, and acceleration. 

Plug and change the three functions and replace the T and angular acceleration and into one function and input the data.

Obtained acceleration a = 0.0366 m/s^2

Using the newton laws and with constant acceleration.


plug the data d= 1 m, a = 0.0366 m/s^2


get the t = 7.4 s.

Uncertainty of time off by 2.7 %.

Conclusion

 The lab is about fining moment of inertia and frictional Torque, and using those data with another experiment to test and check the results which are acceptable.

Uncertainty sources:

1.The Graph of velocity vs time
2. The timer when reading and recording the time.
3. Mistakes made by people during experiments.

May - 05-2015 Lab 16:Angular Acceleration

Lab 16

Purpose: Find a known torque to an object can rotate, and measure the angular acceleration and determine the moment of inertia by using the angular acceleration.

To achieve this, I will do this lab with two parts

Part 1:

Purpose of this part is to collect the basic data such as the weight of object, radius of pulley and disk, and angular acceleration of up and down and average.

Right side is the set up I do on this experiment.
1. Plug the power supply in to the Pasco rotational sensor; make sure that the computer is just reading the top disk.
2. Set up the computer. Open Logger Pro. Choose rotary motion and in option change the counts per rotation to 200
3. Make sure the hose clamp on the bottom is open so that the bottom disk will rotate independently.
4. Turn on the compressed air so that the disk can rotate separetely. Set the disks spinning freely to test the equipment.
5. Start the measurements and release the mass and get the angular velocity vs time graph and get angular acceleration up and down.






To do this correctly, I will do with six different experiments.
1 to 3 are changing the hanging mass from 25g to 75 g and keeping the disk and pulley same.
4 is keeping the 25g mass and disk  and changing the pulley to large one.
5 is keeping the 25 g mass large pulley and changing disk from steel to aluminum
6  is keeping the 25 g mass large pulley and changing disk to two steel disks.

I measure the equipment such as weight of mass and disk, and radius of pulley.

After finish this, I start to do the experiments.
First one:
is 25g mass, 1.36 kg steel disk, and 0.025 m diameter pulley

Second one:
is 50g mass, 1.36 kg steel disk, and 0.025 m diameter pulley

Third one:
is 75g mass, 1.36 kg steel disk, and 0.025 m diameter pulley

Fourth one:
is 25g mass, 1.36 kg steel disk, and 0.0495 m diameter pulley

Fifth one:
is 25g mass, 0.466 kg aluminum disk, and 0.0495 m diameter pulley

Last one
is 25g mass, 0.466 kg steel disk with 1.36 kg steel disk, and 0.0495 m diameter pulley

Here is all the data combine together:

As showing in picture, the angular acceleration up is bigger that angular acceleration down because there is friction on disk. When the mass is going down, the net torque is torque mass - torque friction , so angular acceleration down is smaller than the real. When the mass is going up, the net torque is - torque mass + - torque friction , so angular acceleration up is bigger than the real. Therefore, the real angular acceleration is the average.

Save those data to do next part to calculate the Moment of inertia. In part one, the error or uncertainty are easy to reduce because most of the mistakes are made by people who did this experiments such as the weight and radius. One thing should be reminded is that make sure to change to 200 counts per rotation and spin the disk independently.

Conclusion of Part one:

During part one, I measured the weight of disk and mass, radius of pulley, and angular acceleration for up and down; Seeing from my data, when increasing the hanging mass only, the angular acceleration is increasing as linear curve with a = m + C; when increase the radius of the pulley, the angular acceleration is increasing too as linear with a = r(pulley) + C; when increase the weight of disks, the angular acceleration is decreasing as linear with a = -M(disk) + C.

Part two:

Purpose of this part is to use the data from part one and calculate the moment of inertia by measurement and compare it with moment of inertia by formula and find the friction torque.

To find the moment of inertia

 I need to derive the function by drawing the force.









And using Newtons second and torque with force, angle and moment of inertia set up equation.

Here is the function I used to find Moment of inertia

Because I have done six different experiments



This is first one

Comparing the moment of inertia by using formula and by using measurement, the result I calculated is 3.8% off.

Second:

Comparing the moment of inertia by using formula and by using measurement, the result I calculated is 0.3% off.

Third:

Comparing the moment of inertia by using formula and by using measurement, the result I calculated is 0.339% off.

Fourth:

Comparing the moment of inertia by using formula and by using measurement, the result I calculated is 1.59% off.

Fifth:

Comparing the moment of inertia by using formula and by using measurement, the result I calculated is 0.35% off.

Last:


Comparing the moment of inertia by using formula and by using measurement, the result I calculated is 3.41% off.

Conclusion part two:

Second part of this lab is use the data in part one and derive the moment of inertia function. Plugging the data into the function, I obtained the experiment value (by formula) and original value (by measurement)of disk; the results are under 4% off.
The source of Uncertainty of this lab will be:
One, the data I measured such as weight and radius of disk and pulley have under 5 % off.
Two, when the disk spin, they may not all spin independently.
Three, the equipment may not function perfectly which may cause error two.